Scalar product

The product of two vectors is called scalar product. The result is not a vector but a number (scalar).

$\vec{a}\cdot\vec{b}$ $=\begin{pmatrix}a_1\\a_2\\a_3\end{pmatrix}\cdot\begin{pmatrix}b_1\\b_2\\b_3\end{pmatrix}$ $=a_1 \cdot b_1 + a_2 \cdot b_2 + a_3 \cdot b_3$

Geometric meaning:

$\vec{a}\cdot\vec{b}$ $=|\vec{a}|\cdot|\vec{b}| \cdot\cos(\gamma)$

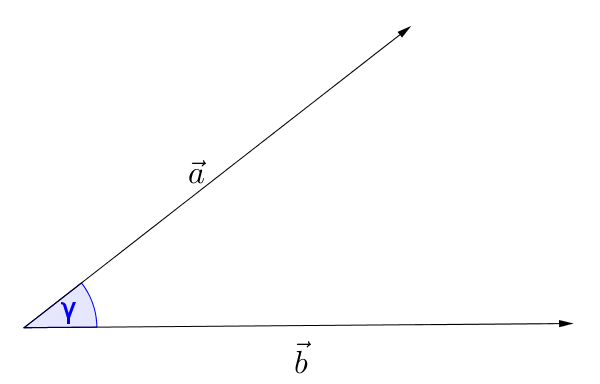
Remember

Two vectors $\vec{a}$ and $\vec{b}$ are only perpendicular if their scalar product equals zero:
$\vec{a}\cdot\vec{b}=0$

Example

$\begin{pmatrix}2\\1\\1\end{pmatrix}\cdot\begin{pmatrix}-2\\3\\4\end{pmatrix}$ $=2\cdot(-2)+1\cdot3+1\cdot4$ $=-4+3+4=3$

Remember

The following calculation rules apply to the scalar product:

Commutative law
$\vec{a}\cdot\vec{b}=\vec{b}\cdot\vec{a}$

Distributive law
$\vec{a}\cdot(\vec{b}+\vec{c})=\vec{a}\cdot\vec{b}+\vec{a}\cdot\vec{c}$

$r\cdot(\vec{a}\cdot\vec{b})=(r\cdot\vec{a})\cdot\vec{b}$ ($r\in\mathbb{R}$)