Math ↠ Vector algebra ↠ Angles between vectors

Angles between two vectors

Using the scalar product you can determine the angle between two vectors.

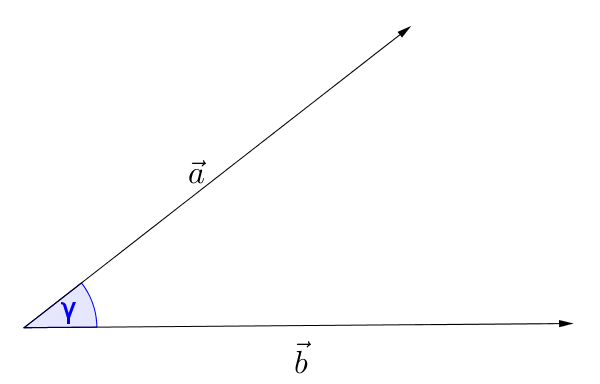
The following formula is used to calculate:

$\cos(\gamma) = \frac{\vec{a}\cdot\vec{b}}{|\vec{a}|\cdot|\vec{b}|}$

$\gamma = \cos^{-1}\left(\frac{\vec{a}\cdot\vec{b}}{|\vec{a}|\cdot|\vec{b}|}\right)$
i

Method

  1. Calculate scalar product
  2. Calculate the vector length
  3. Insert results in the formula

Example

Determine the angle between $\vec{a}=\begin{pmatrix}2\\6\\-3\end{pmatrix}$ and $\vec{b}=\begin{pmatrix}2\\3\\5\end{pmatrix}$.

  1. Calculate the scalar product

    $\vec{a}\cdot\vec{b}$ $=\begin{pmatrix}2\\6\\-3\end{pmatrix}\cdot\begin{pmatrix}2\\3\\5\end{pmatrix}$ $=2\cdot2+6\cdot3-3\cdot5$ $=7$

  2. Calculate the vector length

    $|\vec{a}|=\sqrt{2^2+6^2+(-3)^2}$ $=7$

    $|\vec{b}|=\sqrt{2^2+3^2+5^2}$ $=\sqrt{38}$

  3. Insert results in the formula

    $\cos(\gamma) = \frac{\vec{a}\cdot\vec{b}}{|\vec{a}|\cdot|\vec{b}|}$

    $\cos(\gamma) = \frac{7}{7\cdot\sqrt{38}}$ $= \frac{1}{\sqrt{38}}$

    $\gamma = \cos^{-1}\left(\frac{1}{\sqrt{38}}\right)$ $\approx80.66°$