Extreme value problem
One type of task that uses differential calculus are optimizations or extreme value problems. An extreme value problem is a kind of optimization problem but only with one constraint. It can be both a maximization problem and a minimization problem.
i
Hint
Extreme value problems exist when a target size (e.g., surface area, volume, profit, ...) is to become maximum or minimum. This condition is then the main condition.
!
Remember
In the case of extreme value problems, a function (the objective function) is set up from a main condition and a constraint, whose extreme values are searched for.
i
Method
- Main condition
- Constraint
- Set up objecitve function
- Calculate extreme values of the objective function
- Calculate missing sizes
Example
The largest possible rectangle area has to be limited with an 800 m fence. Calculate the size of both sides and the area.
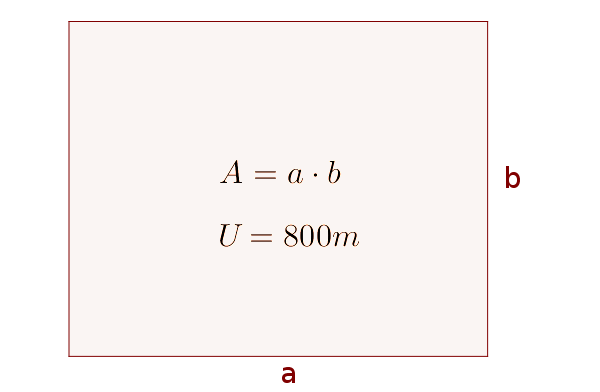
Main condition
The area of the rectangle should be maximized. Therefore, this is the main condition and depends on two variables $a$ and $b$.
$A(a, b)=a\cdot b$Constraint
There are only 800 m of fence available, which limits the area. This is the perimeter of the rectangle.
$P=2a+2b$
$800=2a+2b$Set up objective function
To link both conditions, the constraint is changed to a variable.
$800=2a+2b\quad|-2b$
$800-2b=2a\quad|:2$
$a=\frac{800-2b}2$ $=400-b$
Now this must be used in the main condition and you get the objective function, which is dependent on only one variable.
$A(a, b)=a\cdot b$
$A(b)=(400-b)\cdot b$ $=400b-b^2$-
Calculate extreme values of the objective function
Now you can (as with other functions too) calculate the extreme values of the objective function.
$A(b)=400b-b^2$
$A'(b)=400-2b$
$400-2b=0\quad|-400$
$-2b=-400\quad|:(-2)$
$b=200$
With the second derivation, it is still checked whether the result is actually a maximum point, since the area should be maximum.
$A''(b)=-2$
$A''(200)=-2<0$ => Maximum point -
Calculate missing sizes
$b=200m$
From the (converted) constraint you can now calculate $a$.
$a=400-b$
$a=400-200=200m$
From the main condition (alternatively also with the objective function) the area $A$ can be calculated.
$A(a, b)=a\cdot b$
$A(a, b)=200m\cdot 200m=40.000m^2$
